Mathematics for Meccano
Written by Richard Marsden for our Summer 2016 Newsletter
You’re standing behind your models at the exhibition, discussing the educational aspects of Meccano with some parents. Suddenly their offspring start tugging furiously at their sleeves, anxious to be somewhere else, or indeed, anywhere else. It’s not a school day, and you’ve just used the ‘M’ word. No, not ‘Meccano’, or even ‘money’, but ‘mathematics’. But there is mathematics in Meccano, and the more you look, the more you’ll find. Some children will eventually thrive on this; many don’t. These parents hope theirs will; that’s why they came to the exhibition.
For a start, every Meccano boy and girl might well know his or her nineteen-times table, even if a little hazy about four-times-nineteen and six-times-nineteen.
If they’ve been given more Meccano gear wheels and pinions they can make up ratios of 4:1, 3:1, 2:1 and 1:1. An astute purchase from the inestimable Mr. Taylor will add a first ratio of 5:1. While this is about the right total range for a car gearbox, a car with these ratios would be very awkward to drive, because of the huge gap between 4th and top, especially compared to the much smaller gap between 1st and 2nd. “Why?”, the young constructor might ask. After all, the numbers go up in a steady sequence, 1, 2, 3, 4, 5. An arithmetic series, in fact. No, with regard to the car’s road speed, the series is really 0.2, 0.25, 0.33, 0.5 and 1.0, and that’s a harmonic series, an arithmetic one ‘upside down’. Writing it this way shows the increasing gaps between the gears. What works better is a geometric series, so that changing to the next gear gives the same percentage change in engine speed, regardless of which gear you are moving to.
A typical geometric series might be 0.0625, 0.125, 0.25, 0.5, 1.0, where each number is twice the previous one. In the car gearbox example, the multiplication factor might be 1.5, giving the series approximately 0.2, 0.3, 0.44, 0.67, 1.0. In a real car, for other reasons, the ratios will be spread further in the lower gears and closed up in the higher ones, and top gear does not need to be 1:1, but the point has been made. Three mathematical number series for the price of, well, ten Meccano gear wheels.
Start building structures, and you’ll soon meet Pythagoras’ theorem and wish you hadn’t. It was actually discovered by one of his followers, but Pythagoras, being the boss, took the credit. That’s a lesson in itself. (Strictly it was rediscovered, having been known by at least one earlier civilisation). But back to the mathematics. If you’ve tried bracing a right angle joint with a diagonal strip, you’ll know that most arrangements don’t work. The holes won’t line up. Pythagoras tells you why, but first a quick remark on strip lengths. If we choose our unit to be the half-inch hole spacing, then a 2½” strip counts as 4, № 5. This is because it’s the distance between the centres of the holes that matters, not the overall length of the strip.
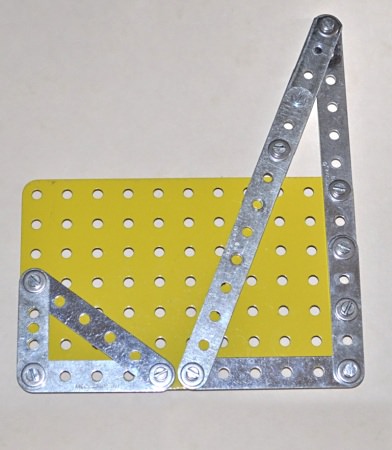
3, 4, 5 and 5, 12, 13 right-angle triangles in Meccano
Pythagoras says that if you multiply each of the two shorter sides in a right-angle triangle by themselves, and add the two answers together, you will get the longest side, also multiplied by itself. So 3, 4, 5 will make a right angle triangle, because 9 + 16 = 25. Annoyingly, it doesn’t work for most combinations of small numbers, hence the holes not lining up. For the few that do, doubling up our example with give you 6, 8, 10, then 9, 12, 15, and so on. There is also 5, 12, 13 and its multiples, then 7, 24, 25 and also 8, 15, 17, but after this the numbers get rapidly too big for practical use (871, 2160, 2329, anyone?). I was always frustrated by the lack of 3” strips in my Meccano set, for the 3, 4, 5 triangle. It took me far too long to notice that if I built a larger model, I could use the more numerous 5½” ones as the diagonals of 6, 8, 10 triangles.
Another consequence of Pythagoras is that you can’t put a bracing strip at exactly 45°. You would need to find two whole numbers one of whose square is exactly half the square of the other one. There’s a simple proof that shows that two such numbers can never be found, no matter how hard you look. You can get near with 12 and 17, closer with 29 and 41, and closer still with 70 and 99. But these latter two would be big triangles, and the 12, 17 combination is not close enough to get a bolt through the final hole, as shown in the photo.
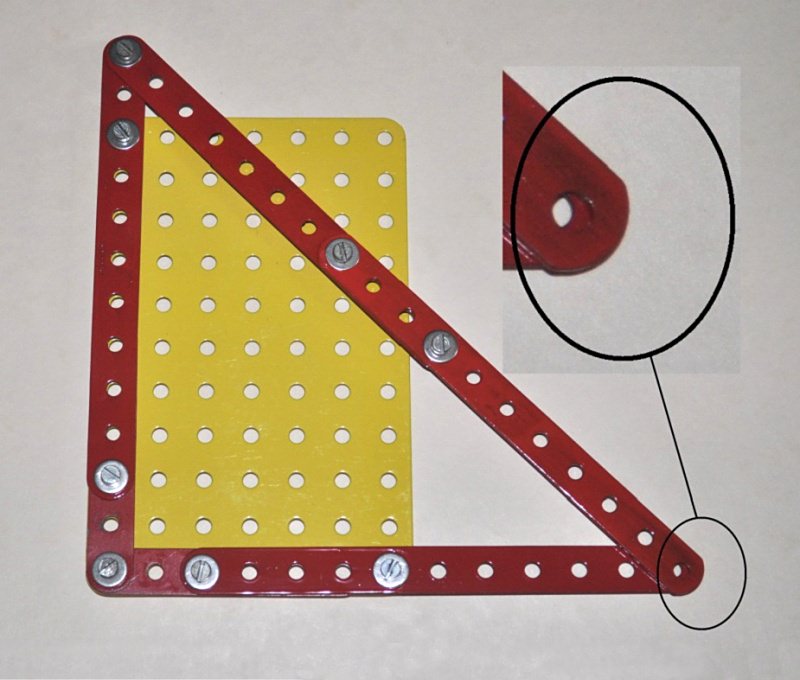
A 12, 12, 17 right-angle triangle in Meccano, showing that the final holes do not quite line up
What this means in mathematical terms is that the square root of two is irrational — it cannot be expressed as a ratio of two whole numbers. In fact the square root of any number that isn’t a ‘perfect square’ such as 4, 9, 16, etc. is irrational. If you tried to write down one of these irrationals exactly, you would never finish. You could always get more accuracy by going to an extra decimal place. Note that this is not the same as recurring decimals such as 0.6666… You can write that as the ratio of 2 to 3.
On another topic, you’ve probably heard of the Golden Ratio. It’s about 0.618:1. A rectangle drawn to these proportions ‘looks right’, useful if building elegant models is your thing. It is said that the ratio occurs frequently in nature. More controversially, if you ask a large number of people to draw a rectangle, it is claimed the results will cluster around the Golden Ratio. I’ve just measured our TV, over the case rather than just the screen, 495mm by 801mm. To three decimal places, that’s 0.618:1. I suspect that’s no coincidence.
The golden ratio
Specifically, if you divide a straight line into two parts, such that the smaller is to the larger as the larger is to the whole line, you’ve generated the golden ratio. It’s the solution to the quadratic equation x2 + x – 1 = 0. But wait a minute, the Fundamental Theorem of Algebra says quadratics have two answers, not just one. Two golden ratios? Don’t worry. You’ll get the answer you expect, approximately 0.618, and another one, approximately minus 1.618. The mathematics has spotted that moving the division point way out beyond the end of the line still gives you the same ratios. Sometimes it’s just too clever!
But why only approximate values? Again, another of those square roots is involved, root five this time. Fortunately, shaving the ratio down to 1.6 gives a nice round number that’s probably close enough. Measure over the entire part rather than between hole centres.
Which brings me round to pi (sorry, terrible pun). In the middle of March you might have seen something in the papers or on the TV declaring it to be pi-day (or π-day). If you wrote the date American-style it was 3/14/16. Or 3.1416. Get it? This is the value, to four decimal places, of the circumference of a circle divided by its diameter. It is the same no matter how large or small the circle, and is denoted by the Greek letter π, equivalent to our letter ‘p’ and pronounced like the thing you eat with chips. You won’t be surprised to hear that π, too, is irrational. This could be one reason why flexible plates gained their slotted end holes, so you can wrap them round 2½” faceplates to make loco boilers. The circumference of a 2½” circle is about 7.85”.
The number π is not just irrational, but goes one better by being transcendental. This has nothing to do with John Lennon, but means it isn’t the solution to any non-zero polynomial equation with rational coefficients. In plain English, it means it will be a total pain to find a way of calculating its value efficiently, should you ever need it to a few trillion decimal places, and you’ll still need to devise a slick computer program to actually perform the calculations within your lifetime. There is at least a simple and direct longhand way of calculating square roots. Not so for π, and some of the first values published, worked out by hand, had errors.
There are several other, more esoteric, mathematical constants such as ‘e’ and ‘gamma’ that are also transcendental, but these don’t have any applications to Meccano as far as I can see. A future Secretary’s Challenge? No, don’t even think it!
But by now the aforementioned offspring have found another child at the exhibition who’s playing Minecraft on an iPad Mini, which is much more interesting. And I haven’t even described how you can use trigonometry and differential calculus to work out the effects of bolts being a slack fit in their holes. Well, we can but hope…